Structural Design of Cantilever Slabs

Cantilever slabs are common features in residential and commercial buildings due to the need to have bigger spaces on upper floors. To achieve this, architects normally extend the slab beyond the ground floor building line, thereby forming a cantilever. Cantilever slabs in reinforced concrete buildings are usually characterised by a reinforced concrete slab projecting from the face of the wall and having a back span that extends into the interior panels of the building.

The design of reinforced concrete cantilever slab is usually governed by serviceability limit state requirements. Typically, the design involves the provision of adequate concrete thickness and reinforcement to prevent excessive deflection and vibration.

During the detailing of reinforced concrete cantilever slabs, the main reinforcements are provided at the top and they extend into the back span by at least 1.5 times the length of the cantilever or 0.3 times the length of the back span, whichever is greater. Furthermore, it is important to provide at least 50% of the reinforcement provided at the top, and at the bottom.

In this article, we are going to show how we can analyse and design cantilever slabs subjected to floor load and block wall load according to the requirements of EN 1992-1-1:2004 (Eurocode 2).

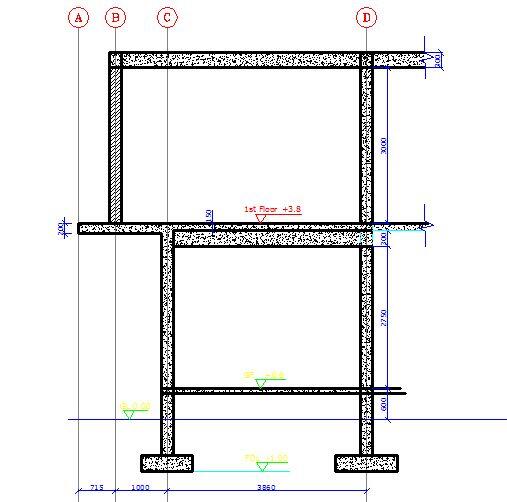
Worked Example – Design of Cantilever Slabs

A cantilever slab 200 mm thick is 1.715m long, and it is supporting a blockwork load at 1.0m from the fixed end. Design the slab using the data given below;

Purpose of building – Residential
f_ck = 25 Mpa
f_yk = 460 Mpa
Concrete cover = 25 mm
Height of block wall = 2.75 m
Unit weight of concrete = 25 kN/m³
Unit weight of block with renderings = 3.75 kN/m²

Load Analysis 

Self weight of slab = (25 × 0.2) = 5 kN/m²
Finishes (assume) = 1.2 kN/m²
Partition allowance = 1.0 kN/m²
Total characteristic permanent action (pressure load) g_k  = 7.2 kN/m²

Permanent action from wall G_k = 3.75  × 2.75 = 10.3125 kN/m

Variable action on slab q_k = 1.5 kN/m²

At ultimate limit state;
n = 1.35g_k + 1.5q_k
n = 1.35(7.2) + 1.5(1.5) = 11.97 kN/m²

Ultimate load from wall = 1.35 x 10.3125 =  13.92 kN/m

Design Forces

M_Ed = (13.92 × 1) + (11.97 × 1.7152)/2 = 31.523 kNm
V_Ed = (13.92) + (11.97 × 1.715) = 34.45 kN

Flexural design

M_Ed  = 31.523 kNm
Effective depth (d) = h – C_nom – ϕ/2 – ϕ_links
Assuming ϕ12 mm bars will be employed for the main bars
d = 200 – 25 – 6 = 169 mm

k = M_Ed/(f_ckbd²) = (31.523 × 10⁶)/(25 × 1000 × 169²) = 0.044

Since k < 0.167, no compression reinforcement required
z = d[0.5+ √(0.25 – 0.882K) ]
z = d[0.5+ √(0.25 – 0.882(0.044))] = 0.95d

A_s1 = M_Ed/(0.87f_yk z) = (31.523 × 10⁶)/(0.87 × 460 × 0.95 × 169) = 490 mm²/m
Provide X12@200 c/c  TOP (AS_prov = 565 mm²/m)

Check for deflection

ρ = A_s,prov /bd = 565 / (1000 × 169) = 0.0033
ρ₀ = reference reinforcement ratio = 10^-3√(f_ck) = 10^-3√(25) = 0.005
Since if ρ ≤ ρ₀;

L/d = K [11 + 1.5√(f_ck) ρ₀/ρ + 3.2√(f_ck) (ρ₀ / ρ – 1)^(3⁄2)

k = 0.4 (Cantilevers)

L/d = 0.4 [11 + 1.5√(25) × (0.005/0.0033) + 3.2√(25) × [(0.005 / 0.0033) – 1]^(3⁄2)
L/d = 0.4[11 + 11.363 + 5.9159] = 11.311

β_s = (500 As_prov)/(f_yk As_req) = (500 × 565) / (460 × 490) = 1.253

Therefore limiting L/d = 1.253  × 11.311 = 14.172
Actual L/d = 1715/169 = 10.147

Since Actual L/d (10.147) < Limiting L/d (14.172), deflection is satisfactory.

Exercise for Students
(1) Provide distribution bars
(2) Verify the section for shear
(3) Check for cracking
(4) Do the detailing sketches

Thank you for visiting Structville today, and God bless.