Design of Beam and Raft Foundation

A beam and raft foundation is a special type of shallow foundation where ground beams are incorporated into the raft slab along the column lines to enhance the structural performance of the slab and to reduce cost. Therefore, the design of a beam and raft foundation involves the provision of adequate member sizes and reinforcement for the ground beams and slab, while ensuring that the bearing capacity of the soil is not exceeded.

In flat raft slab design, punching shear is a major design requirement that influences the thickness of the slab. This often leads to an excessive thickness of slab members that will be economically detrimental to the owner of the building. However, by introducing ground beams along the column gridlines, the problem of punching shear is eliminated as the column bears on the ground beams. This may lead to the use of thinner slabs and bigger heavily reinforced ground beams.

The beams in a beam and raft foundation may be upstand or downstand. The general theory in the design of beam and raft foundations is that the earth pressure intensity as a result of the superstructure load is first resisted by the raft slab, which then transfers the load to the ground beams. Therefore, the uniformly distributed load used in the design of the ground beams is assumed to be transferred from the raft slab.

Since the pressure load on the raft slab is coming from the ground, it follows that the top fibre of the slab is in tension in the sagging areas (span), while the support regions are in tension at the bottom fibre. This is a typical reverse of what is obtainable in the design of suspended solid slabs.

Therefore, for the theory and assumptions made in the design of a beam and raft foundation to be valid, the slab must be in direct contact with the ground, and be stiff enough to resist the earth pressure intensity. This is one of the scepticism of professionals on the use of downstand ground beams in raft foundations.

In the case of down-stand beams, the ground beams are constructed into the natural soil, with a height that rises above the natural ground level, in order to make up the architectural level for the ground floor slab. As a result, the foundation must be filled with imported earth material to the ground beam level, before the raft slab concrete is done.

If the imported material is not properly compacted and eventually settles, the raft slab will behave like a suspended slab and the design philosophy will eventually be defeated. Additionally, the foundation slab will bear on a filled ground with unknown bearing capacity. Therefore, the fill material for raft slabs must be high-quality earth materials (preferably granular materials) and must be properly compacted.

Therefore, upstand ground beams are theoretically more certain since the raft slab bears directly on the natural ground, however, they will be more expensive since another oversite concrete will have to be done. However, down-stand ground beam construction provides an economical advantage to the building owner since the raft slab also functions as the oversite concrete floor.

An Investigation on the Analysis of Beam and Raft Foundation Using Staad Pro

Design Example

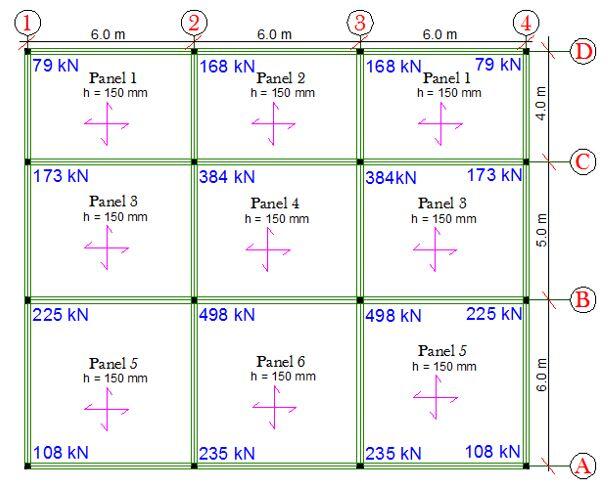
The axial forces in the ground floor columns have been presented below, and have been used to demonstrate how to carry out the design of the beam and raft foundation manually.

Design Data
Allowable bearing capacity of soil = 40 kN/m²
Dimension of all columns = 230 x 230 mm
Dimension of ground floor beams = 1200 x 250 mm
Thickness of ground floor slab = 150 mm
Concrete cover to ground beams = 50 mm (sides and bottom)
Cover to slab = 30 mm
f_ck = 25 MPa
f_yk = 500 MPa

Analysis of the raft slab by rigid approach
A little consideration of Figure 3 will show that the foundation is loaded is symmetrically in the x-direction, therefore we will compute the eccentricity of the load in the z-direction.

Sum of load on gridline D = 79 + 168 + 168 + 79 = 494 kN
Sum of load on gridline C = 173 + 384 + 384 + 173 = 1114 kN
Sum of load on gridline B = 225 + 498 + 498 + 225 = 1446 kN
Sum of load on gridline A = 108 + 235 + 235 + 108 = 686 kN

Resultant of loads R = 686+ 1446 + 1114 + 494 = 3740 kN

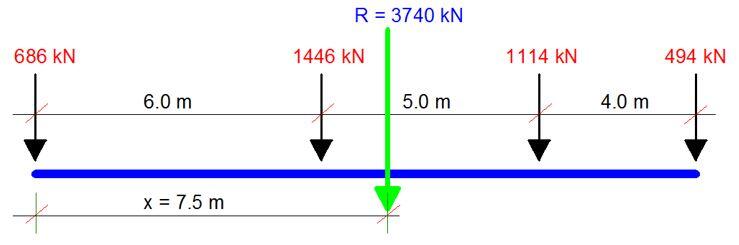
Taking moment about the centroid of the base;

M = (-686 x 7.5) – [1446 x (7.5 – 6)] + [1114 x (7.5 – 4)] + (494 x 7.5) = 290 kNm

Eccentricity of the load e = M/R =290/3740 = 0.0775 m to the right hand side

The base dimensions are taken as follows;
L = 6 + 6 + 6 + 0.25 = 18.25 m
B = 6 + 5 + 4 + 0.25 = 15.25m

Pressure distribution P_i = (R/A) ± (6Re/LB²)

On substituting and solving;
P_max = 13.847 kPa
P_min = 13.028 kPa

These values are way below the allowable bearing capacity of the soil, therefore there is no need to increase the dimensions of the base. Also, due to the close values of P_max and P_min, instead of using a trapezoidal pressure distribution, we can assume a rectangular pressure distribution using the maximum pressure.

Note
Pressure distribution calculation is carried out using SLS load, but we used ULS here. You can convert from ULS to SLS for simple buildings when using Eurocode by using a factor of 1.37 (Ubani, 2017).

Therefore, using a pressure of 13.847 kPa, we can design the raft slabs and the ground beams using the conventional methods which I believe that we are familiar with.

Design of the raft slab

The design of the raft slab is done just like the design of a normal suspended floor, but there is usually no need to check for deflection. For raft foundations without ground beams, punching shear is also very critical.

Self weight of the slab = 25 kN/m² x 0.15 m = 3.75 kN/m²

Design pressure for the raft slab = 13.85 – 3.75 = 10.1 kN/m² (note that it will be improper to factor the self-weight of the ground floor slab since it is favourable in this case)

Effective depth of the slab d = h – C_c – ϕ/2 = 150 – 30 – 10/6 = 115 mm

Where C_c = concrete cover of the raft slab, and ϕ is the diameter of the reinforcement to be used.

General Design Formula

k = M_Ed/(f_ck bd² )
z = d[0.5 + √(0.25 – 0.882k)]
A_s1 = M_Ed/(0.87f_yk z)
A_smin = (0.26f_ctm b_t d)/f_yk ≥ 0.13bd/100

Design of Panel 1

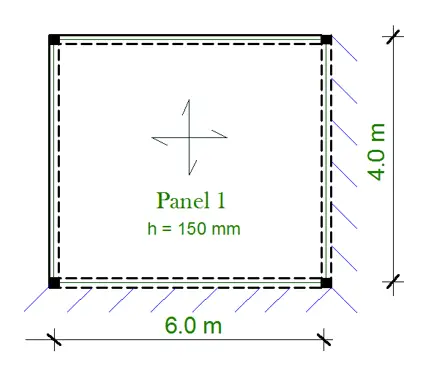
k = l_y/l_x = 6/4 = 1.5

Moment coefficient for two adjacent edges discontinuous

	Short Span	Long Span
Mid-span	0.059	0.034
Continuous edge	0.078	0.045

Short Span – Mid span

M_Ed = 9.5 kN.m
k = 0.028
Since k < 0.167 No compression reinforcement required
z = 0.95d
A_s1 = 193 mm²/m
A_Smin = 153 mm²/m
Check if  A_Smin < 0.0013 b d (187.2 mm²/m)
Therefore, provide H10 @ 250 c/c  TT (A_sprov = 314 mm²/m)

Short Span – Continuous Edge
M_Ed = 12.6 kNm; A_sreq = 254 mm²/m
Therefore, provide H10 @ 250 c/c  BB (A_sprov = 314 mm²/m)

Long Span – Mid span
M_Ed = 5.5 kNm; A_sreq = 127 mm²/m
Therefore, provide H10 @ 250 c/c  NT (A_sprov = 314 mm²/m)

Long Span – Continuous Edge
M_Ed = 7.3  kNm; A_sreq = 161 mm²/m
Therefore, provide H10 @ 250 c/c  TT (A_sprov = 314 mm²/m)

Design of the ground beams

The figure above shows the assumed load distribution from the raft slab to the ground beams. For simplicity of hand calculations, we are going to use the formula for load transfer to the ground beams. This gives unconservative results, especially for shear forces induced in the beam. But can be used for design purposes.

Shorter span = nl_x/3
Longer span = nl_x/3 [1 – 1/3k²]

For the beam on gridline 2

Slab load from Panel 1 and 2
w₁ = w₂ = nl_x/3 = (10.1 × 4)/3 = 13.467 kN/m

Slab load from Panel 3 and 4
w₃ = w₄ = nl_x/3 = (10.1 × 5)/3 = 16.833 kN/m

Slab load from Panel 5 and 6
w₅ = w₆ = nl_x/3 = (10.1 × 6)/3 = 20.2 kN/m

Total load on the spans
w_C-D = 13.467 + 13.467 = 26.93 kN/m
w_B-C = 16.833 + 16.833 = 33.67 kN/m
w_A-B = 20.2 + 20.2 = 40.4 kN/m

The self-weight of the beam in this case is beneficial (favourable), and can be ignored.
We can therefore analyse the ground beam as a continuous beam as follows;

We know that M_A =  M_D = 0 since we are treating it as a simple support.

Let us use the stiffness method for the analysis of the structure. The adopted basic system is shown below.

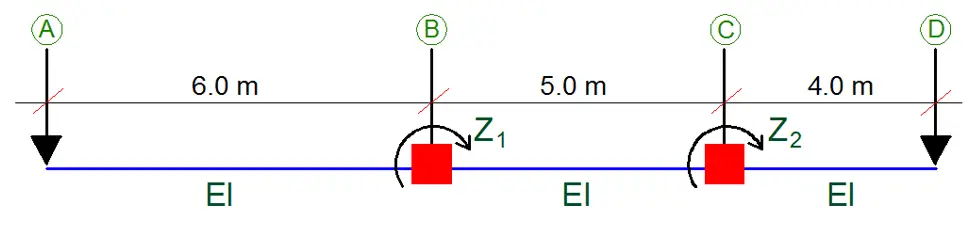
The canonical equation for the beam is given below;

K₁₁Z₁ + K₁₂Z₂ + k_1P = 0
K₂₁Z₁ + K₂₂Z₂ + k_2P = 0

Analysis of Case 1

Z₁ = 1.0; Z₂ = 0

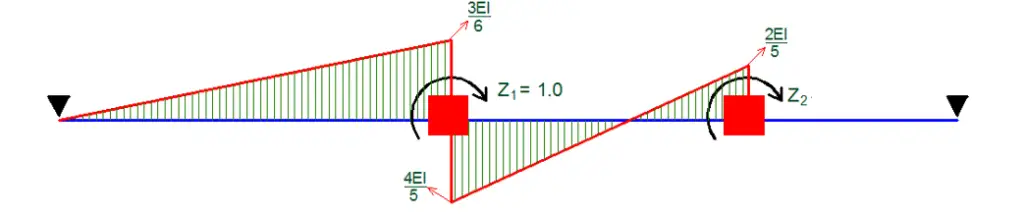
K₁₁ = 3EI/6 + 4EI/5 = 1.3EI
K₂₁ = 2EI/5 = 0.4EI

Analysis of Case 2

Z₁ = 0; Z₂ = 1.0

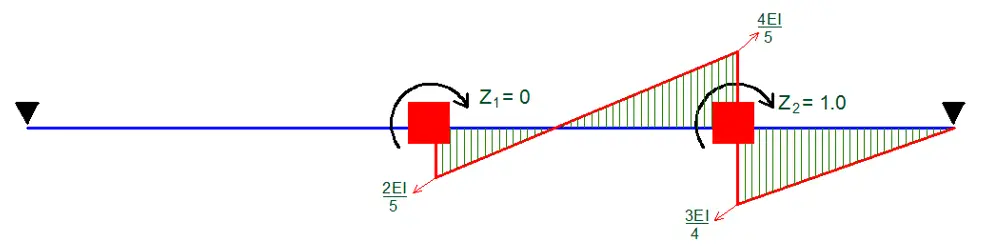
K₂₁ = 2EI/5 = 0.4EI
K₁₂ = 4EI/5 + 3EI/4 = 1.55EI

K_1P = F_BA + F_BC
F_BA = +ql²/8 = (40.5 × 6²)/8 = 182.25 kNm
F_BC = -ql²/12 = (-33.67 × 5^2)/12 = -70.145 kNm
Hence, K_1P = 182.25 – 70.145 = 112.105 kNm

K_2P = F_CB + F_CD
F_CB = +ql²/12 = (33.67 × 5²)/12 = 70.145 kNm
F_CD = -ql²)/8 = (26.93 × 4²)/8 = -53.86 kNm
K_2P = 70.145 – 53.86 = 16.285 kNm

Therefore;
1.3Z₁ + 0.4Z₂ + 112.105 = 0
0.4Z₁ + 1.55Z₂ + 16.285 = 0

On solving;
Z₁ = (- 90.161)/EI radians
Z₂ = 12.761/EI radians

To determine the support moments;
M_i = M_f + M₁Z₁ + M₂Z₂

At support B
M_B^L = 182.25 – [90.161/EI× 3EI/6] = 137.17 kNm
M_B^R = -70.145 – [90.161/EI× 4EI/5] + [12.761/EI× 2EI/5]= -137.17 kNm

At support C
M_C^L= +70.145 – [90.161/EI× 2EI/5] + [12.761/EI× 4EI/5]= 44.289 kNm
M_C^R = -53.86 + [12.761/EI× 3EI/4] = -44.289 kNm

Using the principles of statics, the span moments and shear force diagrams can be drawn.

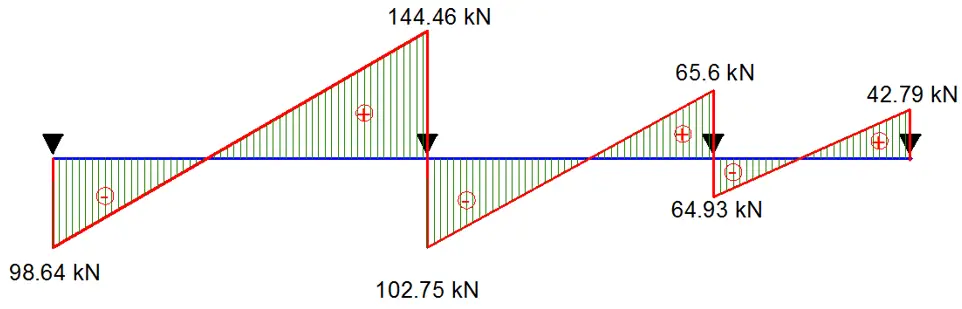
Structural Design

Span A – B
M_Ed = 120.2 kNm

h = 1200 mm
Effective depth d = 1200 – 50 – 10 – (16/2) = 1132 mm (assuming ϕ16 mm reinforcement will be used for the main bars, and ϕ10mm for the links).
Beam width (b_w) = 250 mm

k = M_Ed/(f_ckbd²) = (120.12 × 10⁶)/(25 × 250 × 1132²) = 0.0149
Since k < 0.167 No compression reinforcement required

z = d[0.5 + √(0.25 – 0.882k)] = d[0.5 + √(0.25 – 0.882(0.0149)] = 0.95d

A_s1 = M_Ed/(0.87f_yk z) = (120.12 × 10⁶)/(0.87 × 500 × 0.95 × 1132) = 257 mm²

To calculate the minimum area of steel required;
f_ctm = 0.3 × f_ck^2⁄3 = 0.3 × 25^2⁄3 = 2.5649 N/mm² (Table 3.1 EC2)

A_Smin = 0.26 × f_ctm/f_yk × b_t × d

Where b_t is the width of the beam in tension. A little consideration will show that the flange of the beam is in tension. If we are to use the flange of the beam the way it is in tension,

A_Smin = 0.26 × 2.5649/500 x 2290 x 1132 = 3457 mm²

In BS 8110-1:1997, the minimum area of steel required when the flange is in tension is;
A_smin = (0.26b_wh)/100 = (0.26 ×250 ×1200)/100 = 780 mm²

Eurocode experts have suggested using the area of the T-section for the calculation of the minimum reinforcement
Such that the A_Smin = 0.26 × f_ctm/f_yk × A_c
A_c = (2290 x 150) + (1050 x 250) = 60600 mm²
A_Smin = 0.26 × 2.5649/500 x 60600 = 819 mm² (this is acceptable).

Therefore provide 5H16 Top (A_sprov = 1005 mm²)

Design of support B
MEd = 137.71 kNm
Note that at support B, the web is in tension and we will need to consider the flange width in the calculation of the area of steel required.
l₀ = 0.15 (l₁ + l₂) = 0.15(6000 + 6000) = 1800 mm
b₁ = b₂ = (6000 – 250)/2 = 2875 mm
l₀ = 0.85l₁ = 0.85 x 6000 = 5100 mm

b_eff,1 = b_eff,2 = (0.2 x 2875) + (0.1 x 1800) = 755 mm which is not less than 0.2l0 = (0.2 x 1800) = 360 mm
Hence, b_eff,1 = b_eff,2 = 360 mm < 755 mm
b_eff = 250 + 360+ 360 = 970 mm
k = M_Ed/(f_ck bd² ) = (137.71 × 10⁶)/(25 × 970 × 1132²) = 0.00443
Since k < 0.167 No compression reinforcement is required

z = 0.95d

A_s1 = M_Ed/(0.87f_yk z) = (137.71 × 10⁶)/(0.87 × 500 × 0.95 × 1132) = 294 mm²
A_Smin = 0.26 × 2.5649/500 x 250 x 1132 = 377 mm²
Check = 0.13bd/100 = (0.13 × 250 ×1132)/100 = 367.9 mm²
Therefore provide 2H16 Bottom (Asprov = 402 mm²)

On looking at the internal stresses diagram, this reinforcement provided can go all the way in the beam.

Shear Design
Using the maximum shear force for all the spans
Support B; V_Ed = 144.46 kN
V_Rd,c = [C_Rd,c.k. (100ρ₁ f_ck )^1/3 + k₁.σ_cp]b_w.d ≥ (V_min + k₁.σ_cp) b_w.d

C_Rd,c = 0.18/γ_c = 0.18/1.5 = 0.12

k = 1 +√(200/d) = 1 +√(200/1132) = 1.42 > 2.0, therefore, k = 1.42

V_min = 0.035k^(3/2) f_ck^(1/2) = 0.035 × (1.42)^(3/2) × (25)^(1/2) = 0.296 N/mm²

ρ_1 = As/bd = 402/(250 × 1132) = 0.00142 < 0.02;

σ_cp = N_Ed/A_c < 0.2f_cd (Where N_Ed is the axial force at the section, A_c = cross-sectional area of the concrete), f_cd = design compressive strength of the concrete.) Take N_Ed = 0

V_Rd,c = [0.12 × 1.42(100 × 0.00142 × 25 )^1/3] 250 x 1132 = 73564 N = 73.564 kN
Since V_Rd,c (73.564 kN) < V_Ed (144.46 kN), shear reinforcement is required.

The compression capacity of the compression strut (V_Rd,max) assuming θ = 21.8° (cot θ = 2.5)
V_Rd,max = (b_w.z.v₁.f_cd)/cot⁡θ + tanθ
V₁ = 0.6(1 – f_ck/250) = 0.6(1 – 25/250) = 0.54
f_cd = (α_cc f_ck)/γ_c = (0.85 × 25)/1.5 = 14.167 N/mm²
Let z = 0.9d

V_Rd,max = [(250 × 0.9 × 1132 × 0.54 × 14.167)/(2.5 + 0.4)]× 10^-3 = 671.896 kN
Since V_Rd,c < V_Ed < V_Rd,max
Hence Asw/S = V_Ed/(0.87f_ykzcot θ) = (144.46 × 10³)/(0.87 × 500 × 0.9 × 1132 × 2.5 ) = 0.13

Minimum shear reinforcement;
Asw/S = ρ_w,min × b_w × sinα (α = 90° for vertical links)
ρ_w,min = (0.08 × √f_ck)/f_yk = (0.08 × √25)/500 = 0.0008
A_sw/S_min = 0.0008 x 250 x 1.0 = 0.2
Since 0.200 > 0.13, adopt 0.2 as the minimum shear reinforcement

Maximum spacing of shear links = 0.75d = 0.75 × 1132 = 849
Provide Y8mm @ 300mm c/c as shear links (A_sw/S = 0.335) Ok

Download the full calculation sheet from the link below;
Manual Design of Beam and Raft Foundation to Eurocode 2 [PDF]

References
Ubani O.U. (2017): Practical Structural Analysis and Design of Residential Buildings Using Staad Pro V8i, CSC Orion, and  Manual Calculations. Ist Edition, Volume 1. Structville Integrated Services

To download the above-named textbook, click on the image below;