Comparative Analysis of Triangular Pile Caps | Free PDF download

When the most economical number of piles that will satisfy design requirements is three, a triangular-shaped pile cap can be adopted. In this case, the piles are arranged in a triangular manner, and equidistant from each other (usually spaced at 3 times the diameter of the pile).

Following the same arrangement, a similarly shaped pile cap can be used to transfer the loads to the piles. Triangular pile caps can be analysed and designed using the strut-and-tie method, beam analogy, or finite element analysis.

In our post on Structural Aspects of Pile Foundation Design, we showed how we can determine the number of piles required to support a column load, and we went ahead to design a triangular pile cap based on the strut-and-tie method (truss analogy).

In this article, we are going to analyse the same triangular pile cap using bending theory and finite element analysis and compare the results obtained.

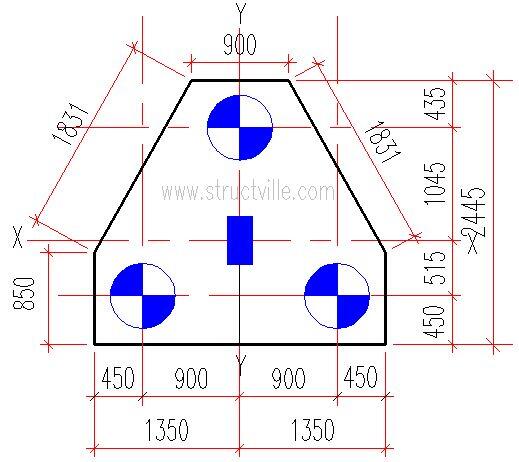
The layout of the pile cap is shown in Figure 1 and we are going to assume that it is subjected to an axial load of 2500 kN from a 300mm x 300mm column (neglecting the orientation of the column in Figure 1).

Bending Theory Analysis of Triangular Pile Cap

The load on each pile is computed based on its distance from the centroid of the column

Load on the single pile
N₁ = N(A_y – A_y‘)/A_y
Where:
N = Axial load from column = 2500 kN
A_y = Vertical distance between the centriod of piles in the y-direction = 515 + 1045 = 1560 mm
A_y‘ = Vertical distance between the centroid of the column to the centroid of the single pile in the y-direction = 1045 mm

Hence N₁ = 2500 x (1560 – 1045)/1560 = 825. 321 kN

Load on the twin piles
N₂ = (N – N₁ ) / 2
N₂ = (2500 – 825.321)/2 = 837.3395 kN

Bending moment in the x-direction
M_x = N₂ x (A_x – C_x)/2
A_x = Spacing of the twin piles = 1800 mm
C_x = Width of the column parallel to x-direction = 300 mm

M_x = 837.3395 x (1.8 – 0.3)/2 = 628 kNm

Bending moment in the y-direction
M_y = N₁ x (A_y‘ – C_y/2)
A_y‘ = Spacing of the twin piles = 1045 mm
C_y = Width of the column parallel to y-direction = 300 mm
M_y = 825. 321 x (1.045 – 0.3/2) = 738.662 kNm

Finite Element Analysis of Triangular Pile Cap

The pile cap was modelled as a 1300 mm thick concrete plate element on Staad Pro, while the piles were modelled as 600 mm diameter columns with a length of 2m supported on a fixed base. The short column was adopted to avoid wide variation of result due to second-order effects and to avoid large displacement which is not very practicable and also not considered in manual analysis.

The results obtained from Staad Pro are given in Figures 3 – 6 below;

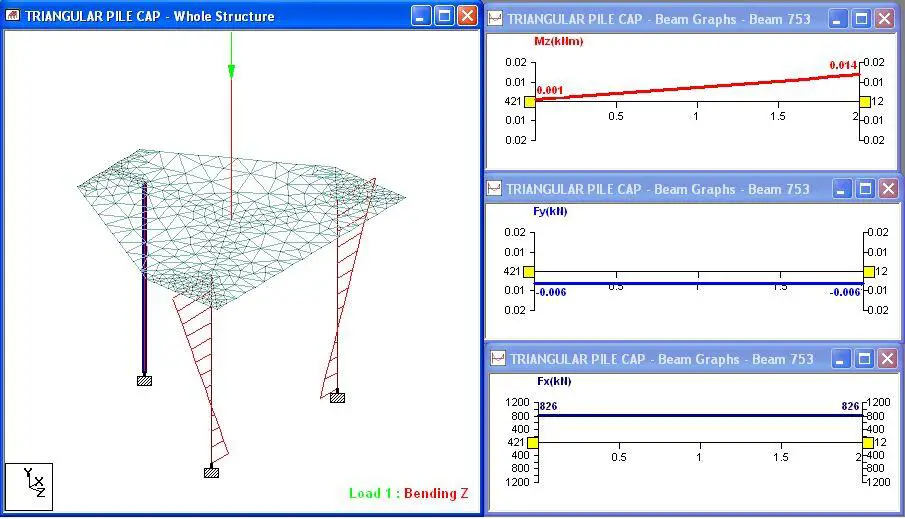
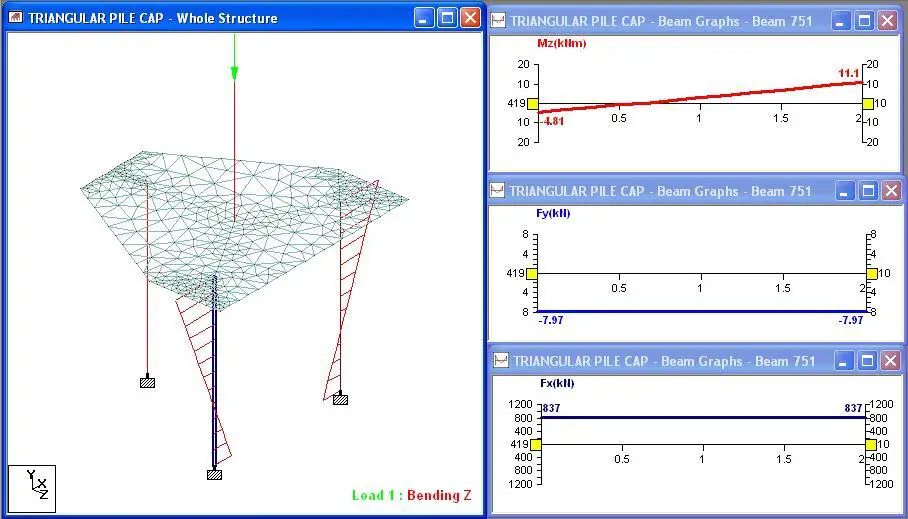
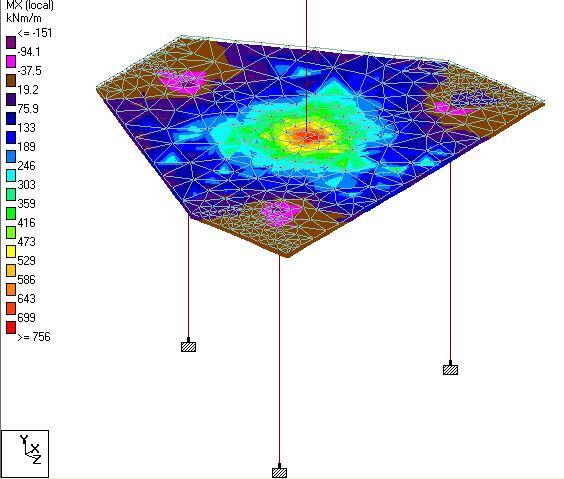
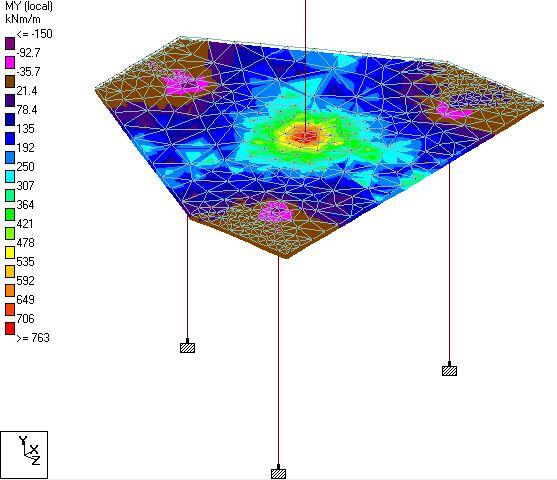
The summary of the results between manual analysis (bending theory) and Staad Pro (finite element analysis) is shown in Table 1.

Action effect	Bending theory	FEA (Staad Pro)	Percentage difference
Single pile load (kN)	825.32	825.77	0.054%
Twin pile load (kN)	837.32	837.12	0.024%
Mx (kNm)	628	756.07	18.507%
My (kNm)	738.66	762.904	3.22%

From Table 1, it could be seen that the distribution of load to the piles was found to be approximately the same for both approaches, but a wide variation was observed for M_x bending moment between the two methods. The M_y bending moment was found to be close with a percentage difference of about 3.229%.

Therefore, Staad Pro can be used to model triangular pile caps and the result can be used for design purposes. The result obtained from this study is open to discussion, especially based on the difference obtained in bending moment values. We will be glad to hear from you!

To download the full structural analysis and design calculation sheet, click HERE