Design of Bridge Pier and Pier Cap

Bridge pier caps are horizontal structural members used for transferring bridge deck loads to the piers, before they are transferred to the foundation. They can be made of different shapes or forms, depending on the required aesthetics of the bridge. However, pier caps (or heads) must be designed to resist the actions transferred from traffic, self weight of the bridge deck, wind actions, or accidental actions due to vehicle impact. By the nature of their configuration, the piers and the pier cap/head of bridges should be designed as monolithic rigid frames.

However, some of the different configurations that are obtainable in bridge piers are;

• Solid wall pier
• Hammer head bridge pier
• Rigid frame bridge pier

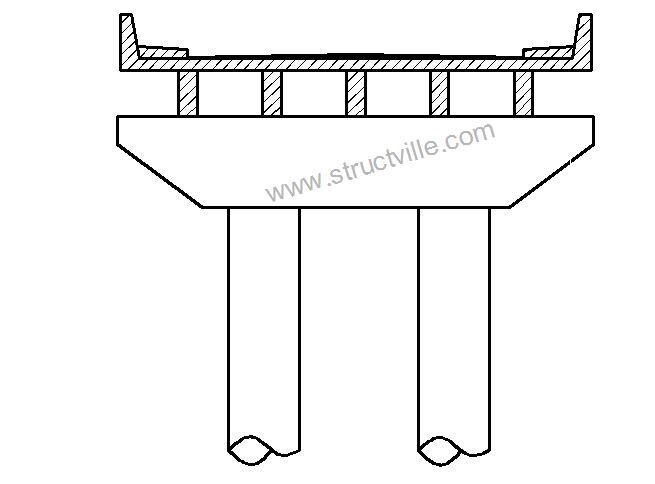
In this article, we are going to show how to design reinforced concrete rigid frame bridge pier and pier cap using Staad Pro software. Different methods can be used for obtaining the reactive forces from the bridge deck such as grillage analysis, finite element analysis, or classical methods such as Courbon’s theory. We will pick the reactive forces from finite element analysis, and apply them to the bridge pier cap.

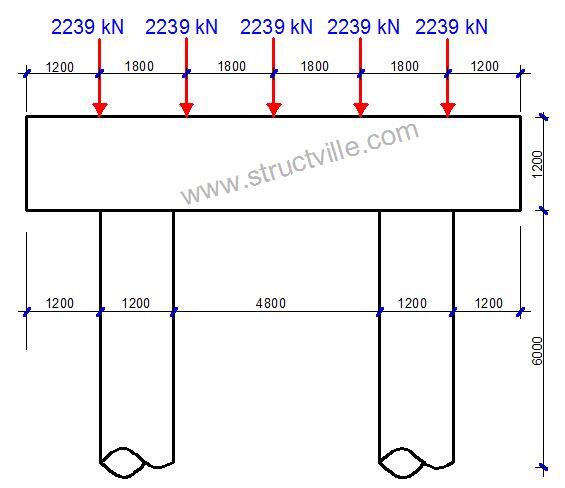
The configuration of the bridge deck is shown below;

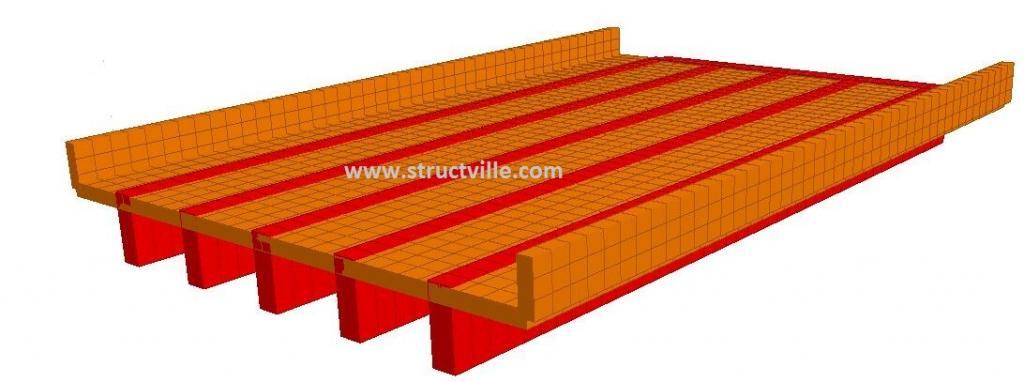
The length of the bridge deck is 15 m, and the loading and configuration is shown below;

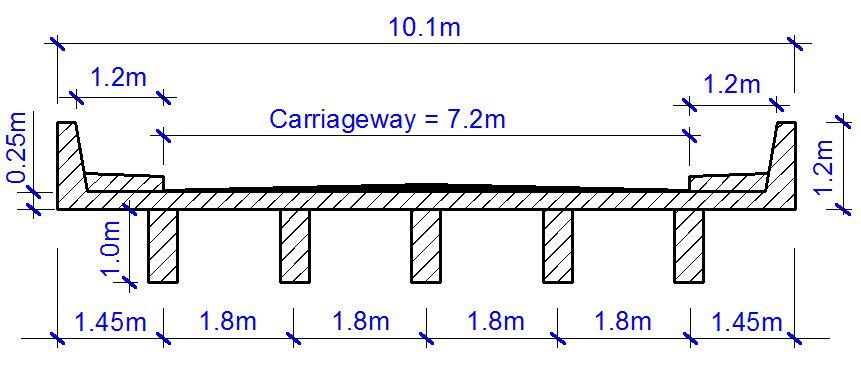
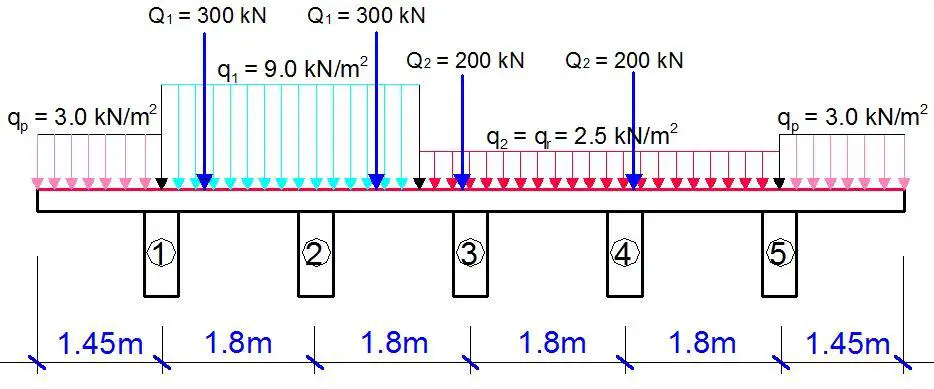
The maximum reactive forces from finite element analysis are as follows;

Self weight = 276.288 kN
Traffic UDL = 194.839 kN
Traffic moving load = 320.762 kN

These reactions are transmitted through the elastomeric pad bearing to the pier cap. Note that there are accompanying horizontal actions due to braking, acceleration forces, skidding of vehicles etc. Design value of braking or acceleration forces depend on the vertical loads applied on notional lane 1. The values of horizontal forces should be combined with appropriate value for vertical traffic load corresponding to its frequent value. However, when the leading traffic loads are vertical ones, the accompanying value of the braking and acceleration forces are to be defined in the National Annex and can be set to zero. But for simplicity, horizontal actions have not been considered in this article.

Therefore the ultimate load that will be transferred to the pier cap is given by;

V_Ed = 1.35G_k + 1.5Q_k = 1.35(276.288) + 1.5(194.839 + 320.762) = 1146.39 kN

Since this is assumed to be an intermediate pier, the pier cap will be subjected to double of the load above. Therefore, the maximum ULS load from each girder will be 1146.39 x 2 = 2239 kN. Note that the factored self weight of the pier and pier cap should be included in the model.

Fro proper analysis and design of the foundation, it is important to separate the service and ultimate loads properly. At SLS, the maximum reactive force from each girder is 791.889 kN. The preliminary sizing of the rigid frame pier cap and the design data of the pier system is shown below;

Diameter of piers = 1200 mm
Dimensions of pier cap = 950 x 1200 mm
Support of frame = Fixed

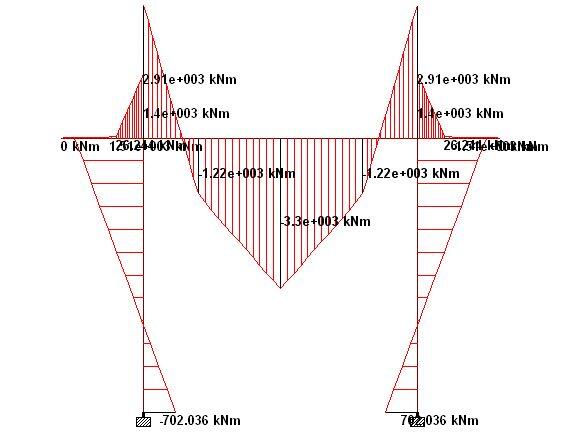
When analysed on Staad Pro, the internal forces in the rigid frame pier cap are as follows;

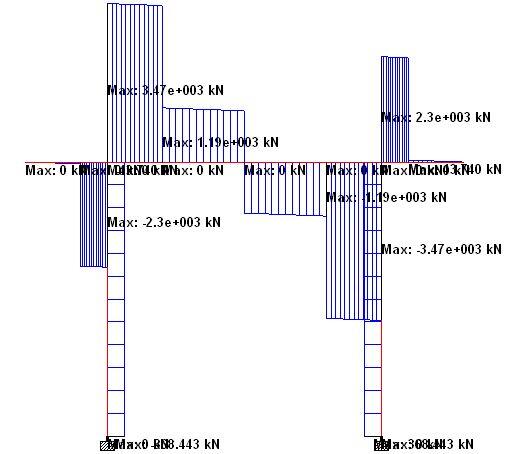
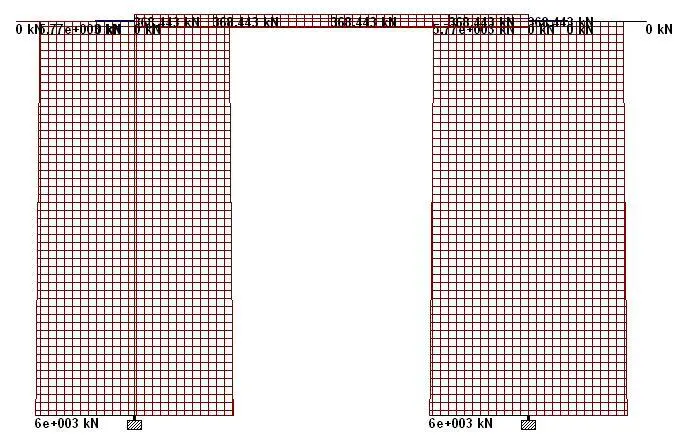
The summary of the internal forces at ULS are as follows;

Pier Cap
Sagging moment = 3298.257 kNm
Hogging moment = 2911.468 kNm
Maximum shear force = 3468.85 kN
Maximum axial force = 368 kN (compression)

Piers
Maximum moment = 1508.62 kNm
Maximum shear force = 368.443 kN
Maximum axial force = 6001.483 kN

Structural design of the Pier Cap

Let cover to reinforcements = 50 mm
Yield strength of reinforcement f_yk = 500 MPa
Compressive strength of concrete f_ck = 40 MPa

Span – Sagging

M_Ed = 3298.257 KN.m

Effective depth (d) = h – C_nom – ϕ/2 – ϕlinks
Assuming ϕ25 mm bars will be employed for the main bars, and ϕ12 mm bars for the stirrups (links)
d = 1200 – 50 – 12  – 12 = 1126 mm

k = M_Ed/(f_ckbd²) = (3298.257 × 10⁶)/(40× 950 × 1126²) = 0.0684

Since k < 0.167, no compression reinforcement required
z = d[0.5+ √(0.25 – 0.882k) ]
z = d[0.5+ √(0.25 – 0.882(0.0684))] = 0.935d

A_s1 = M_Ed/(0.87_yk z) = (3298.257 × 10⁶)/(0.87 × 500 × 0.934 × 1126) = 7209 mm²
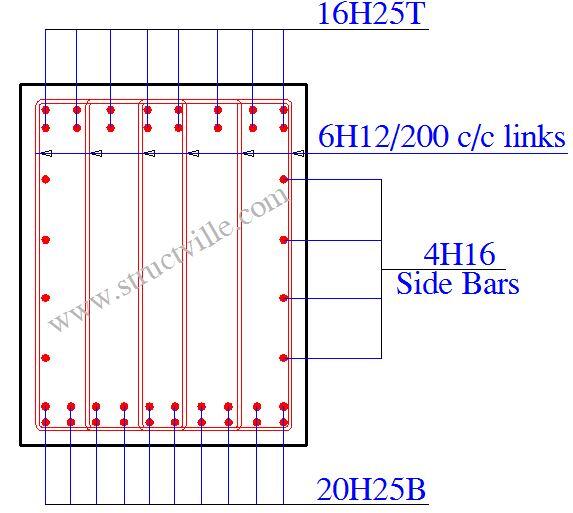
Provide 20H25mm BOT (AS_prov = 8380 mm²)

Hogging
M_Ed = 2911.468 KN.m

k = M_Ed/(f_ckbd²) = (2911.468 × 10⁶)/(40 × 950 × 1126²) = 0.0604
Since k < 0.167, no compression reinforcement required
z = d[0.5+ √(0.25 – 0.882K)]
z = d[0.5+ √(0.25 – 0.882(0.0604))] = 0.943d

A_s1 = M_Ed/(0.87f_yk z) = (2911.468 × 10⁶)/(0.87 × 500 × 0.943 × 1126) = 6303 mm²
Provide 16H25mm TOP (AS_prov = 6704 mm²)

Shear Design (Support A)
V_Ed = 3468.85 kN
N_Ed = 368 KN (compression)

We are going to anchor the 16No of H25mm reinforcement provided fully into the supports.

V_Rd,c = [C_Rd,c.k.(100ρ₁ f_ck)^(1/3) + k₁.σ_cp]b_w.d

Where;
C_Rd,c = 0.18/γ_c = 0.18/1.5 = 0.12
σ_cp = N_Ed / bd = (368 × 1000) / (950 × 1126) = 0.344 N/mm²
k = 1 + √(200/d) = 1 + √(200/1126) = 1.42> 2.0, therefore, k = 1.42
ρ₁ = As/bd = 6704/(950 × 1126) = 0.00627 < 0.02; K₁ = 0.15

V_Rd,c = [0.12 × 1.42(100 × 0.00627 × 40 )^(1/3) + (0.15 × 0.344)]  × 950 × 1126 =  588745.259 N = 588.745 KN

Since V_Rd,c (588.745 KN) < V_Ed (3468.85 KN), shear reinforcement is required.

The compression capacity of the compression strut (V_Rd,max) assuming θ = 21.8° (cot θ = 2.5)

V_Rd,max = (b_w.z.v₁.f_cd)/(cot⁡θ + tanθ)
V₁ = 0.6(1 – f_ck/250) = 0.6(1 – 40/250) = 0.504
f_cd = (α_cc ) f_ck)/γ_c = (1 × 40)/1.5 = 26.667 N/mm²
Let z = 0.9d

V_Rd,max = [(950 × 0.9 × 1126 × 0.504 × 26.667) / (2.5 + 0.4)] × 10^-3 = 4461.811 kN

Since V_Rd,c < V_Ed < V_Rd,max
Hence, A_sw/S = V_Ed/(0.87f_ykzcot θ) = 3468850/(0.87 × 500 × 0.9 × 1126 × 2.5) = 3.147

Minimum shear reinforcement;
A_sw/S = ρ_w,min × b_w × sinα (α = 90° for vertical links)
ρ_w,min = (0.08 × √(f_ck))/f_yk = (0.08 × √40)/500 = 0.001012
A_sw/S (min) = 0.001012 × 950 × 1 = 0.961

Maximum spacing of shear links = 0.75d = 0.75 × 1126 = 844.5 mm
Provide 6H12mm @ 200mm c/c (A_sw/S = 3.39) Ok

Note that this link must be properly closed with adequate anchorage length, because it will assist in resisting torsion.

Side Bars
Provide H16@200 c/c

The typical detailing of the pier cap is shown below. Note that bar marks and layer information were not included.

Design of the piers

You are expected to go through the process of designing columns according to Eurocode 2. You will need to check if the column is short or slender. If the column is slender, you will need to obtain the additional moments due to second order effects.

However for simplicity in this post, let us add the effect of imperfection to the column moment. You can go through the rigourous process of ensuring that you are designing for the worst effect.

Let us assume that effective length of the pier is 0.85L = (0.85 x 6000) = 5100 mm (note that Eurocode requires a more rigourous approach for calculating the effective length of columns)

The geometric imperfection of the column e_i = [(1/200) x (5100/2)] = 12.75 mm

e_iN_Ed = 6001.483 x 0.01275 = 76.5189 kNm

Let us therefore take the column design moment M_Ed to be = 1508.62 + 76.5189 = 1585.13 kNm

Hence,
N_Ed = 6001.483 kN
M_Ed = 1585.13 kNm
d = 1200 – 50 – 50 = 1100
d/h = 0.9 (say)

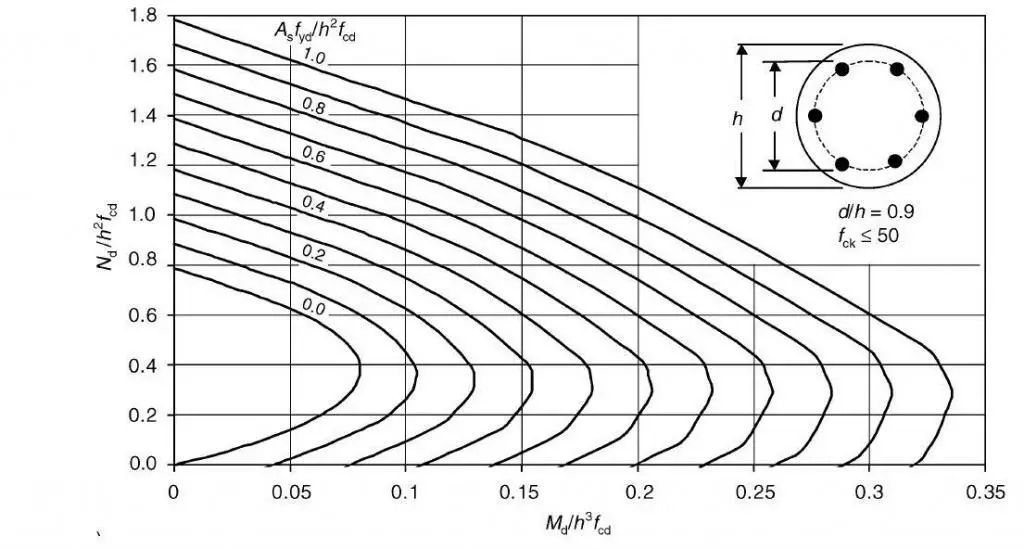
Let f_cd = (0.85 x 40)/1.5 = 22.667 N/mm²

N_Ed/h²f_cd = (6001.483 x 1000)/(1200² x 22.667) = 0.183 (Conservatively say 0.2)
M_Ed/h³f_cd = (1585.13 x 10⁶)/(1200³ x 22.667) = 0.04

Therefore, A_sf_yd/h²f_cd = 0.1
A_s.req = (0.1 x 1200² x 22.667)/(0.87 x 500) = 7504 mm²

The minimum area steel required is 0.1N_Ed/f_yd = 1379 mm² or 0.002Ac = 2262 mm² whichever is greater.

Therefore provide 20H25 (A_sprov = 8380 mm²)

To determine the shear reinforcement, shear design should be carried out. The reinforcement provided should be used to check the pier against vehicle collision. Note that this accidental load case should be unfactored and considered to be acting alone.