How to Load R.C. Columns for Maximum Design Moment

Previously, we have made a submission on how to calculate column axial loads by considering beam support reactions. Just in case you missed it, you can read it by following the link below. But this post highlights the methods of loading columns in a building in order to obtain the maximum design moment.

Read…
How to Calculate Column Axial Load By Considering Beam Support Reactions

Columns in buildings may be axially, uniaxially, or biaxially loaded. An axially loaded column is a column that is subjected to axial load only. This type of column usually exists in the centres of a building where bending moments from floor beams will normally neutralise each other. When a column is subjected to a bending moment in one direction, then it is uniaxially loaded, and when it is subjected to bending in both directions, it is biaxially loaded.

Consider the general arrangement of a building that is as shown above;

Column A1 is a biaxially loaded column because the load from beam 1:A-B is generating a bending moment in the x-direction, while the load from beam A:1-2 is generating a moment on the column in the z-direction. This moment is usually in the form of a fixed end moment, which is finally distributed to the other members meeting at that node based on their stiffness.

Column B1 is a typical example of a uniaxially loaded column. The bending moments from beams 1:A-B and 1:B-C will typically neutralise each other, while the load from beam B:1-2 will exert a bending moment in the z-direction. Typical examples of axially loaded columns in the building are columns B2 and C2.

When a structural design is to be carried out manually, the design engineer has to determine the bending moment on columns as appropriate, alongside the axial loads.

However, while it has been pointed out above that column B1 is uniaxially loaded due to the beams on gridline 1-1 neutralising each other, have you considered a situation whereby PANEL 1 is fully loaded with stored materials, while PANEL 2 is empty?

This situation is very possible during the service life of the structure, and as a result, the column at that instance will not behave as uniaxially loaded, but as biaxial. This is just to give us a little idea of what may influence our loading while carrying out such an analysis. The consideration to be made during designs is usually the worst possible load regime.

Reynolds and Steedman (2005) gave us a good idea of how to approach some aspects of this issue. Loading all the spans at the ultimate limit state (say 1.35g_k + 1.5q_k) will seldom give us the maximum design moment. However, alternating the loads (say one span 1.35g_k + 1.5q_k and the other span 1.0gk) will give us an unbalanced moment, which is a possible worst scenario in practice.

So I am going to give us a rundown of the methods that we can use to load structures (sub-frames) in order to determine the maximum bending moment on columns.

Method 1: Full Subframe

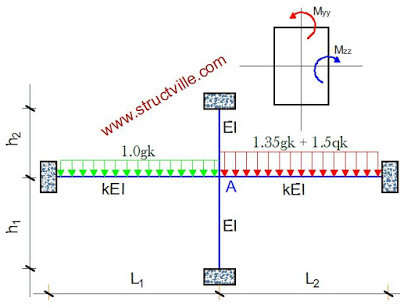
Let us consider the image above showing the sub-frame of a building with the intent of determining the maximum design moment of the lower column at joint C. What we have to do is to load 1.0g_k and (1.35g_k + 1.5q_k) on the spans adjoining span C such that the unbalanced moment is maximised.

In this case, it is preferable to apply 1.35g_k + 1.5q_k on the longer span which is CD, and 1.0gk on the shorter span BC. While this is more representative, the setback is that you will have to solve a 5 x 5 simultaneous equation using the stiffness method of structural analysis before arriving at your answer and hence may not be very handy for a simple scientific calculator process.

Method 2

In this case, the sub-frame is simplified as shown above, but it works best if span BC is longer than the adjoining spans. If span BC is shorter than the adjoining span, you can switch to the use of method 3. Here, we apply a load of 1.35g_k + 1.5q_k on span BC, and 1.0g_k on span AB and CD.

We only solve a 2 x 2 simultaneous equation when using this method, and it is very convenient for classroom and simple design purposes. Realise also that we have to reduce the stiffness of the adjoining beams by half because we are actually overestimating the actual stiffness of the beam by considering all ends to be fully fixed.

Method 3

In this case, we are going to load (1.35g_k + 1.5q_k) on the longer span, and 1.0g_k on the shorter span such that the unbalanced moment at C is maximum. We are still going to reduce the stiffness of the beam by half for the same reasons stated in method 2 (see how to calculate the stiffness of beams).

The advantage of this method is that we are going to solve for just one unknown (rotation at point C) in order to obtain the design moment in question. This is the most popular approach that is taught in most classrooms and utilised for simple designs or checks. I have written some MATLAB programs for carrying out such analysis.

After loading the column, we can now analyse the structure completely by using the stiffness method (my recommendation). An example of this has been done in the next post, and you can take a look by clicking the link below;

Read…
How to Analyse Sub-Frames For Column Design Moment

Thank you for visiting Structville Today. Ensure you follow the link above for the full package of this post.