Many civil engineering projects usually involve excavating into water-bearing soil formations. Before beginning such excavations, the proper system(s) for managing and controlling groundwater and surface water run-off should be planned. This can only be done in practice if you are aware of the ground and groundwater conditions you are likely to face through site investigation data.

It is necessary to take precautions to manage groundwater flows and pore water pressures in water-bearing soils in order to prevent problematic circumstances during excavation and construction. Effective management of surface water runoff is also necessary. Understanding the potential effects of an excavation can help determine which groundwater control measures are required to assure stability.

There are three groups of methods available for temporary works control of groundwater:

(a) Lowering groundwater levels in construction by means of water abstraction, in other words – groundwater lowering or dewatering.
(b) Exclusion of groundwater inflow to the area of construction by some form of very low permeability cut-off wall or barrier (e.g. sheet-piling, diaphragm walls, artificial ground freezing).
(c) Application of fluid pressure in confined chambers such as tunnels, shafts and caissons to counterbalance groundwater pressures (e.g. compressed air, earth pressure balance tunnel boring machines).

Groundwater Control Techniques

Techniques for the control of groundwater can be divided into two principal types:

(a) Those that exclude water from the excavation (known as exclusion techniques)
(b) Those that deal with groundwater by pumping (known as dewatering techniques)

Exclusion Methods of groundwater control

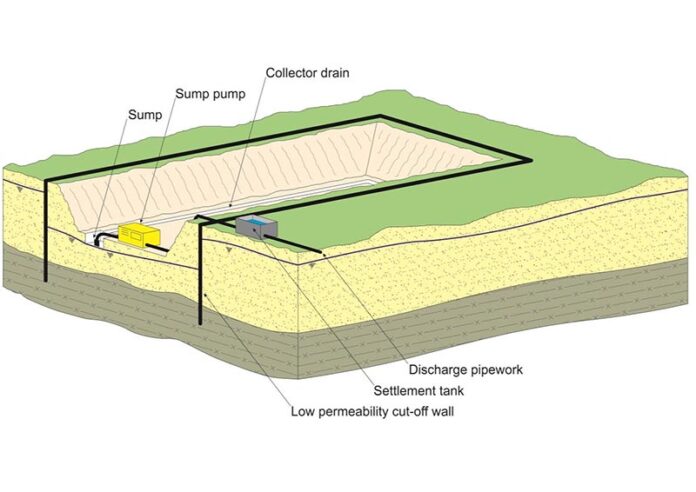
The aim of groundwater control by exclusion is to prevent groundwater from entering the working area. Creating a physically impermeable cut-off wall or barrier around the perimeter of the excavation to keep groundwater out is one of the most widely used exclusion methods. The cut-off often produces a basal seal for the excavation by penetrating vertically down to a very low permeability stratum.

The depth and make-up of any underlying permeable stratum have a significant impact on the costs and viability of constructing a physical cut-off wall. Base instability is possible when upward seepage occurs beneath the base of the cut-off wall due to the lack of or presence of a sufficient very low permeability layer. In these situations, dewatering techniques and exclusion techniques may be combined.

As an alternative, a horizontal barrier or “floor” might be formed adjacent to the cut-off structure to stop vertical seepage. Although it is not common, horizontal barriers have been built utilizing techniques such jet grouting, mix-in-place grouting, and artificial ground freezing.

A portion of the groundwater will become trapped inside the working area if a complete physical cut-off is established. In order to move further with the project, this must be removed, either by sump pumping during excavation or by pumping from wells or wellpoints beforehand.

The capacity of the exclusion technique to enable work to be carried out below the groundwater level with little impact on groundwater levels outside the site is one of its attractive features. This ensures that any groundwater-lowering problems are prevented. Exclusion techniques are frequently employed instead of dewatering techniques, especially in metropolitan areas, to reduce the danger of settlement damage brought on by reducing groundwater levels.

However, it is crucial to remember that practically all walls will leak to some degree when considering the use of the exclusion technique to prevent groundwater level from dropping in areas beyond the site. Particularly vulnerable to leakage are any joints (between columns, panels, piles, etc.) left behind from the installation process.

Several issues can arise if groundwater leaks through cut-offs into the excavation or work area:

(i) The seepages may hinder site operations during construction, necessitating the deployment of sump pumps or surface water management techniques to keep the working area dry.
(ii) The risk of settlement or other negative impacts may result from the leaking into the excavation being severe enough to locally lower groundwater levels outside the site.
(iii) If the cut-offs are a permanent construction, like the walls of a deep basement, even tiny seepages over time will be ugly and could interfere with any architectural finishes that have been applied to the walls.

Grouting or other treatments can frequently be used to address the major seepages that cause issues (i) and (ii). On the other hand, it might be quite challenging to stop or stop the little seepages of (iii). Costs significantly increase if a completely dry or leak-proof construction is required. If cut-off walls are going to be included in the permanent works, this is something that needs to be taken into account.

The techniques used in groundwater exclusion are listed below;

Steel Sheet Piling

In this groundwater control technique, steel sheet piles are driven into the soil to form a barrier against the intrusion of groundwater into the construction area. This is one of the most prominent techniques used in the construction of cofferdams. This technique is applicable to most open soils, however, it can be challenging when obstructions such as rock boulders are encountered.

Steel sheet pile walls may be installed to form permanent cut-off, or used as temporary cut-off with piles removed at the end of construction. They offer the advantage of rapid installation and are relatively cheap. Additionally, they can support the sides of the excavation with suitable propping.

The disadvantages are that the seal may not be perfect, especially if obstructions are encountered. Vibration and noise of driving may be unacceptable on some sites, but ‘silent’ methods are available.

Vibrated beam wall

In this method, a grout injection nozzle is driven into a specially made wide flange beam section using a vibratory driver-extractor that is attached to the beam’s base. A self-hardening slurry is injected into the ground with the designed beam while it is vibrated into the ground to act as a lubricant.

When the beam is withdrawn, the extracted beam element leaves a minimum of a 4 to 6-inch panel that is filled with the self-hardening slurry. A continuous cutoff wall is created by the consecutive beam element insertions and the overlapping of the earlier beam insertions.

The Vibrating Beam construction technology enables operations in small spaces with little room for above-ground mixing or staging. This method also decreases soil disposal costs, which can be expensive when dealing with hazardous locations, as less excavation is needed. Permeabilities in the range of 10-8 cm/sec are capable of allowing for depths greater than 50 ft.

This method is applicable in open excavations in silts and sand but will not support the soil.

Slurry trench cut-off wall

A slurry cut-off wall or slurry trench wall is an excavation made deep into the ground while simultaneously pumping an engineered slurry mix into the trench. A permanent low permeability barrier to groundwater and leachates is created by the slurry cut-off wall after it is completed. Slurry trench cut-off walls also have the capacity to prevent the transportation/movement of a range of heavy metals and organic contaminants including volatile organic compounds (VOCs), hydrocarbons, diesel, solvents and tar.

The slurry trench walls create a low permeability curtain wall surrounding the excavation and it is a permanent water exclusion system. Slurry trench walls are can be quickly installed and relatively cheap, but the cost increases rapidly with depth. It is very applicable in silts, sands, and gravel up to a permeability of about 5 x 10^-3 m/sec.

Structural Concrete Diaphragm wall

Diaphragm walls can be used as cut-off walls for dams or excavation pits, as foundations, or as enclosures for structures. A diaphragm wall is a structural concrete wall constructed panel by panel in a deep trench excavation using either precast or in-situ concrete pours.

Diaphragm walls can serve as retaining walls, water-cut-off structures, deep foundations, basement walls, or as separating structures for underground facilities. They are constructed as ground-level concrete or concrete reinforced with steel walls. They are thought to be almost water-impermeable and deformation-resistant.

Diaphragm walls are permanent structures that support the sides of the excavation and often form the sidewalls of the finished construction. They can be keyed into rock and have the advantage of minimum noise and vibration. However, high cost may make the method uneconomical unless walls can be incorporated into a permanent structure.

It is applicable in side walls and shafts in most soils and weak rocks but the presence of boulders may cause problems.

Secant (interlocking)and contiguous bored piles

A retaining wall made of closely spaced bored piles can be used to construct a deep basement or a cut-and-cover tunnel. The piles could be constructed so that they almost touch each other (contiguous). A watertight retaining wall can be created by grouting the spaces between the piles.

This method of construction results in a more effective form of structure when the piles interlock. The piles will typically need propping during soil excavation before the permanent floor and/or roof structure is finished.

Jet Grouting

In jet grouting, a stabilizing fluid is injected into the subsoil (or the soil being treated) under high pressure and high velocity. The high-velocity fluid jets are used to create different geometries of cemented soil in the ground and typically form a series of overlapping columns of soil/grout mixture that can prevent the movement of groundwater.

Jet grouting can be messy and create large volumes of slurry. There is a risk of ground heave if not carried out with care. Jet grouting is Relatively expensive and applicable for open excavations in most soils and very weak rocks.

Deep soil mixing columns

In this permanent water exclusion solution, overlapping columns are formed by in-situ mixing of soil and injected grout/cement using auger-based equipment. Soil mixing can be categorised into deep soil mixing and shallow soil missing. The mixture of binding agent and natural soil produces columns with very low permeability. This approach produces little spoil, and it is less flexible than jet grouting. It is relatively expensive.

Injection grouting using cementitious grout

Injection grouting is a process by which grouts are injected under pressure into open fissures, voids, cracks, and pores in a soil/rock mass. The grout fills the pore spaces, preventing the flow of water through the soil.

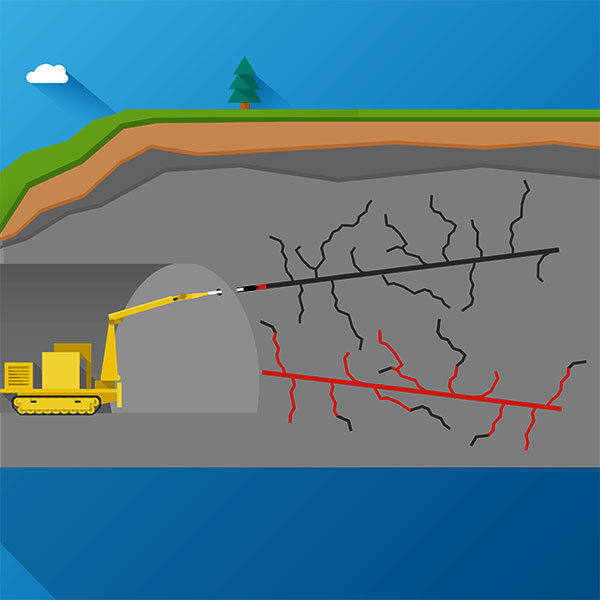
Equipment is simple and can be used in confined spaces. A comparatively thick zone needs to be treated to ensure a continuous barrier is formed. Multiple stages of treatment may be needed. The procedure is applicable to tunnels and shafts in gravels and coarse sands, and fissured rocks.

Artificial ground freezing

Ground freezing is a temporary water exclusion solution during construction. In this solution, a ‘wall’ of frozen ground (a freeze wall) is formed using brine or liquid nitrogen, which can support the side of the excavation as well as exclude groundwater.

This approach may not work if groundwater flow velocities are excessive (> 2 m/day for brine or 20 m/day for liquid nitrogen). Liquid nitrogen is expensive but quick; brine is cheaper but slower. Liquid nitrogen is to be preferred if groundwater velocities are relatively high. Plant costs are relatively high.

Compressed air

In this temporary groundwater control solution, increased air pressure (up to 3.5 bar) raises pore water pressure in the soil around the chamber, reducing the hydraulic gradient and limiting groundwater inflow. Potential health hazards to workers. Air losses may be significant in high-permeability soils. High running and set-up costs.

It is suitable for confined chambers such as tunnels, sealed shafts and caissons.

The level of risk associated with foundation or slab movement at a site is determined by the expansion potential of soil or sedimentary bedrock formation. Any soil or rock element that has the ability to expand in volume with increasing water content is often referred to as expansive soil.

The volume of soil decreases as a result of consolidation, which is a process that forces water out of the pores and fills them with soil particles. However, during swelling, water is absorbed into the soil, which is the reverse of consolidation, in that it forces the soil’s particles apart and causes an increase in volume. This swelling may result in issues very similar to differential settlement by lowering the soil’s ultimate bearing capacity and shear strength.

Swell and swelling pressure can result from elastic restitution after a load is removed, from water adhering to the surface of soil particles, or from the particles expanding as a result of the adsorption of water into the soil particles. Of these, the adsorption of water is the most common. To comprehend the phenomenon of adsorption, a basic understanding of clay mineralogy is required.

The soil structure, dry density of the soil, the initial moisture content, and the availability and characteristics of water are all factors that affect swelling properties in addition to soil composition.

Effect of Soil Structure on Expansion Potential of Soil

The way soil particles interact will depend on their orientation within the soil mass and their distance from one another. The particles may obtain different degrees of orientation depending on the circumstances present during the deposition.

The particle orientation for flocculated and dispersed soil structures is shown in Figure 2. The figure exemplifies the extremes of totally flocculated and fully dispersed soil structures. Particle orientation in most soils would fall between these two extremes. It is convenient to think about the structures that are exhibited while considering the effects of density and soil structure on expansion potential.

It is obvious that the interactions between micelles in a flocculated structure, such as the one in Figure 2a, are principally driven by the contacts between the ends of the particles and the faces of adjacent ones. In contrast to the dispersed structure, the flocculated structure has a greater gap between particles.

It follows that in contrast to the dispersed structure shown in Figure 2b, crystalline and osmotic swelling would be less effective in the flocculated structure. The soil structure would lean more toward the dispersed structure for highly overconsolidated clays that have been subjected to substantial overburden stresses.

Effects of Water Content on Expansion Potential

Swelling won’t occur until there is free water available. Studies have revealed that depending on the type of ions in the water and whether or not there are ions already adsorbed on the soil particles, the electrolyte concentration of the water may change the swelling characteristics. The soil’s expansive properties could be significantly changed if the ions in the water have the capacity to replace the ions that have been adsorbed there.

The expansion potential will also be influenced by the distance between particles, the hydration states of the cations, and particle orientation. As a result, it would be expected that soil with a high dry density and low initial water content would have a higher expansion potential than soil with a lower dry density and a higher initial water content.

Chen (1973 and 1988) conducted oedometer experiments on samples that had been compacted to the same initial density but with different initial water contents. The results of the findings are shown in Figure 3. It is obvious that the initial water content significantly influenced the percentage swell.

Chen (1973 and 1988) also performed oedometer tests on samples with different dry densities and the same initial water content. Figure 4 shows these findings for percentage swelling and swelling pressure. They demonstrate quite clearly that both the percentage swelling and the swelling pressure were significantly influenced by the initial density. As a result, the expansion potential of the soil increases with initial soil moisture content and soil density.

Effects of Dry Density on Expansion Potential

The expansion potential for a remoulded soil sample increases with the density to which the sample is compacted. Similar to this, the swell potential decreases as water content increases.

Dry density is one of these parameters that is crucial. This is explained by taking into account how many soil particles there are in a given volume. The surface area accessible for the adsorption of water will increase with the number of particles in a unit volume, increasing the potential for swelling.

It was observed during laboratory studies that when the degree of compaction increases, swelling in the presence of water at a certain confining pressure increases. This can be attributed to the fact that more clay particles would occupy the same volume due to the greater compaction.

Studies with compacted clays have shown that soils compacted on the dry side of the optimum moisture content are likely to have a flocculated structure, whereas soils compacted on the wet side of optimum moisture content tend to have a dispersed structure, suggesting that expansion potential may be attributable to soil structure. Although soil structure may contribute to the mechanisms causing swelling, it’s possible that the initial moisture content at which the soil is compacted is more significant.

The capacity of the soil to absorb water is closely correlated with swelling potentials. It is clear that swell potential depends on the initial moisture content because the mass can only absorb a given amount of water. Compared to samples of the same density compacted wet of optimum, samples compacted dry of optimum exhibit higher swelling characteristics and swell to larger water contents.

Geotechnical engineering is a branch of civil engineering concerned with the engineering behaviour of soils. Geotechnical engineers apply the principles of soil and rock mechanics to the design of the foundation of all structures and infrastructures resting on the ground, and the use of geomaterials (soils and rocks) for construction. They are also interested in ground improvement, and protection of the soil and groundwater from pollution. Geotechnical engineering is also known as soil engineering.

Geotechnical engineers, therefore, design the foundations of buildings, bridges, dams, embankments, highways, earthworks and deep excavations,  natural and man-made earth slopes, landfills, retaining walls, tunnels, cofferdams, etc. In practice, they work closely with structural engineers, highway/transportation engineers, geologists, water resources engineers, etc.

Soil is a product of nature with so many different properties. Some soils are firm and stiff enough for construction purposes, while some soils are weak and marginal, hence unsuitable for construction purposes.  

The loads from all structures are ultimately transferred to the ground, and it is expected that the ground should be able to withstand the load without undergoing excessive settlement or shear failure. Furthermore, when geomaterials are to be used in civil engineering constructions such as highways, embankments, earth dams, etc, the material selected should have specific engineering properties that will prevent premature failure of the construction. It is the job of geotechnical engineers to recommend suitable materials or design the stabilisation/modification of existing materials.

During the design of foundations, the size and type of foundation can be altered to ensure that the pressure on the soil is within allowable limits. This is essentially the job of geotechnical engineers.

In some cases, the load coming from the structure may be too high, or the soil near the surface may be too weak, such that the geotechnical engineer may recommend a deep foundation such as piles. Using the geotechnical soil investigation report, geotechnical engineers recommend the depth, size, type, and allowable load on the pile. 

In essence, geotechnical engineers perform geotechnical analysis to assess site condition, perform field and environmental investigations, plan and conduct geotechncial exploration, review construction design proposls and approve geotechnical aspects.

A lot of theories are applied during the design of foundations and other earthen structures, and a geotechnical engineer combines them with soil investigation findings to decide on the most suitable foundation. 

Some important topics of geotechnical engineering are;

(1) Shear strength of soils
(2) Compressibility and consolidation
(3) Stresses in soil
(4) Phase relationship and physical properties of soils 
(5) Compaction
(6) Characterisation/Classification of soils

These topics form the basis of many geotechnical engineering design concepts such as foundation design, retaining walls design, stability of slopes, dam engineering, highway construction etc. Other important topics in geotechnical engineering are the flow of water through soils (hydraulic conductivity), soil dynamics, ground improvement, etc.

Geotechnical engineers are also interested in the improvement of marginal soils such as expansive soils, collapsible soils, etc in order to improve their engineering properties. 

Depending on the findings of the geotechnical soil investigation report, overexcavation and replacement of the weak soil with a more suitable material may be recommended. Other solutions can include, stabilisation of the soil with cement, lime, pozzolans, agricultural and industrial wastes, etc. Fibres and geotextiles are also used in soil stabilisation and improvement.

Other methods such as the use of vertical sand drains, deep soil mixing, stone columns, dynamic compaction, micro piles, and grouting can be used to densify weak soils and reduce settlement potential. 

Therefore, the practice of geotechnical engineering relies heavily on soil investigations which usually include laboratory and field testing of soil and rock samples. The results obtained from laboratory investigations are then used to carry out design with an appropriate factor of safety.

Geotechnical engineers are found in private consultancy, in academia, as borehole experts, construction industry, the oil and gas industry, the mining industry, military and warfare, water resources companies, etc. Their services can include; foundation design, design of earthen structures, groundwater extraction consultancy, verification of designs, design of machine foundations, structural design of highway pavements and embankments, ground improvement, etc.

Geotechnical engineers usually have civil engineering as their first degree, and may go ahead to obtain post graduate degrees in geotechnical engineering. They must also be registered/licensed to practice engineering in their country or state. This involves passing all the professional exams and interviews involved in the qualification process.

Some of the aspects of geotechnical engineering that are distinguished as a result of research and practice are as follows:

• Transportation geotechnics
• Geo-environmental engineering 
• Energy geotechnics
• Rock mechanics
• Soil mechanics
• Foundation Engineering 
• Marine/offshore geotechnics

Professional Bodies in Geotechnical Engineering

Some notable geotechnical engineering professional bodies are;

• International Society of Soil Mechanics and Geotechnical Engineering (ISSMGE)
• Academy of Geo-professionals
• Canadian Geotechnical Society (CGS)
• Chinese Institution of Soil Mechanics and Geotechnical Engineering China Civil Engineering Society  (CISMGE)
• Indian Geotechnical Society (IGS)
• Japanese Geotechnical Society (JGS)
• Kazakhstan Geotechnical Society (KGS)
• Korean Geotechnical Society (KGS)
• Mexican Society of Geotechnical Engineering (SMIG)
• Russian Society of Soil Mechanics and Geotechnical, and Foundation Engineering (RSSMGFE)

Since ancient times, people all over the world have practised the art of strengthening earthen structures with brushwood, bamboo, straw, and other materials of a similar nature. The additional strength added by such reinforcements gave birth to mechanically stabilised earth (MSE) walls and structures.

In the year 1963, a French architect and engineer named Henri Vidal was successful in obtaining a patent for a general configuration of applying the aforementioned principle to the construction of embankments. It was adopted for retaining walls, bridge abutments, dams, foundations, and several other applications on a global scale.

The following three fundamental elements make up the patented general configuration, as shown in Figure 1.

1. The earthfill (often chosen granular materials passing US sieve no. 200 with less than 15%).
2. Soil reinforcement. Currently, this takes the shape of metal strips, geotextiles, or wire grids that are attached to the facing unit and extend enough into the earth backfill. To reduce the length of the anchorage length required, special end anchorages can also be offered.
3. The facing unit (this is usually made of metal or concrete blocks made to maintain an aesthetic appearance of the structure and prevent soil erosion).

The Mechanism of MSE Wall

The friction generated at the soil reinforcement interfaces to stop the relative motion of the soil and reinforcement is an important component of the MSE mechanism. The reinforcements provide an apparent cohesion proportionate to the density and tensile resistance of the reinforcement and limit the lateral deformation of the reinforced earth mass.

Depending on the type of structure and the loading conditions, the greatest tensile force in the reinforcement occurs at a distance from the mechanically stabilised earth wall. The reinforced earth is separated into the two zones shown below along this line of maximal tensile force;

1. An active zone behind the facing where the shear forces are directed outward giving rise to an increase of tensile forces in the reinforcements.
2. A resistant zone, where the shear stresses are mobilized to prevent the sliding of the reinforcement which is directed towards the free end of the reinforcement inside the embankment.

Full-scale experiments and observations show that the behaviour of reinforced earth walls is quite different from that of classical retaining walls. The locus of the maximum tensile force in the reinforcement has been found to be different from Coulomb’s failure plane.

The remarkable features of mechanically stabilized earth (MSE) are the following:

1. Strength – It can resist significant earth pressure and seismic force.
2. Flexibility – They are flexible gravity structures. It adapts to substandard foundation soils and large settlements. (This is one of its main advantages over rigid walls.)
3. Construction – It can be easily constructed by untrained labour.
4. Low costs – Costs are low.
5. Aesthetic factors – It has good appearance as the facing can be made of attractive designs.

Design of Mechanically Stabilised Earth Walls

Mechanically stabilised earth walls should be designed against the following types of failures:

1. Tension failure of the reinforcement in the earth (internal stability)
2. Bearing capacity failure of the base (external stability)
3. Sliding of the whole block ABCD along the base (external stability)
4. Overturning and tilting under the horizontal earth pressure acting on the mass.

Usually, the effect of surcharge in concentrated load is assumed to be distributed using the 2 vertical to 1 horizontal distribution as in Figure 3.

The following two types of design methods are used for determining the tension in reinforcements:

1. The working stress method
2. The failure plane method (limit state method).

In the working stress method, we assume the surface of maximum tension in the reinforcement based on experimental values and work out the necessary anchorage length required for the soil reinforcement. It is used as a general case to deal with all types of reinforced earth walls.

In the failure plane method, we consider the equilibrium of several wedges along a potential failure plane and estimate the tension to be developed in the steel reinforcement. We then design for maximum tension. As the working stress method is the more popular and general method, we will discuss only this method in this chapter. The conservative Coulomb’s failure surface is also assumed as the surface of failure plane.

Reinforcement Design of Mechanically Stabilised Earth Wall

The design procedure is as follows for a retaining wall without any surcharge:

Step 1: Adopt vertical and horizontal spacing of the reinforcement to be used. It may range from 0.2 to 1 m vertically and 0.7 to 1m horizontally.

Step 2: Assume the locus of maximum stress in the soil reinforcements. Different authorities have suggested different locus as shown in Fig. 26.2. The most commonly used is the conservative Coulomb’s failure plane.

Step 3: Determine the magnitude of horizontal pressure at various depths due to earth pressure as well as due to any superload on the embankment. Usually, the active earth pressure distribution is assumed and the pressure due to superload may be approximately evaluated by using 2 vertical to 1 horizontal distribution.

Step 4: Take each reinforcement strip and determine the maximum tension that will be developed in it. Let it be T_i. Thus, take the reinforcement strip at depth z from the top. The friction f developed will depend on the pressure on the strip and the coefficient of friction f = γztanδ.

If b is the width of the strip assuming that friction acts on both faces, the anchorage length La required to anchor T_i will be as follows:

L_a = T_i/2bf = T_i/(2bγztanδ)

where;
T_i = tension in soil reinforcement
b = breadth of the reinforcement
γz = pressure at depth z
f = friction developed between soil and reinforcement = tan δ
Ti = (γgK_A) × (area of influence of reinforcing strip)

For each strip, find L_a, the anchorage length required to develop the corresponding anchorage.

(Note: In the field, for easiness of fabrication and installation, all the strips are made of the same length equal to the required maximum anchorage length.)

Soil Reinforcement Selection

It is important to choose the MSE reinforcement carefully. Low-creep materials are necessary for rigid structures like retaining walls. Materials with some creep may be advantageous for embankments that may consolidate over time. Nonmetallic reinforcements are weaker than metallic reinforcements like galvanized steel and stainless steel (used to reduce corrosion).

Corrosion resistance is a benefit of plastics. Plastics come in both fabric and nonfabric forms. Fabrics are created by weaving or knitting textiles. Grids and strips make up non-fabrics; the latter is occasionally strengthened with glass fibre. In any case, these reinforcements should be chosen only after a thorough examination of their strength, creep, and durability properties. Based on the outcomes of laboratory tests provided by the manufacturer or by accredited laboratories, they should base their decision.

Reference:
Varghese P. C. (2012): Foundation Engineering. PHI Learning Private Limited, New Delhi

Filters are essential for earthen constructions such as dams to be protected against seeping groundwater. A lot of literature on geotechnical engineering contains a number of empirical design criteria for filters that were created based on experimental research and prior engineering knowledge.

In the past, earth layers of various gradations or sizes were predominantly used to create filters. However, geotextile filters are increasingly widely used because of their low cost and relatively simple construction.

Applications of Filter Materials

An impermeable or permeable wall can be used to enclose the excavation in saturated soil. The pressure on an impermeable wall can be two to three times higher than on a permeable wall, on which only the effective earth pressure is acting.

On the contrary, both the earth pressure and the water pressure act on an impermeable wall. The pressures on the wall can be reduced by lowering the water level behind it, such as through pumping wells or drainage into the excavation pit. However, the in-situ soil surrounding the excavation is subjected to a hydraulic gradient as a result.

For internally unstable soils, the flow forces generated by this hydraulic gradient might result in the transport of fine soil particles into the skeleton of coarse soil particles. Unless the surface is protected with a filter and drainage material, the soil can erode at the surface where the water exits the soil body, such as at the pumped drainage well or at other drainage locations.

Whenever there is an impounded reservoir, a hydraulic gradient and water pressure are imparted to the soil foundation. The effective stresses and resulting soil strength are decreased by the elevated hydrostatic water pressure. The soil particles are subjected to flow forces by the imposed hydraulic gradient, which can lead to erosion at the soil body’s surface or within the soil’s skeleton, where water seeps out of the ground.

The erosion of soil particles will be stopped, and the effective stress will be raised, by adding a layer of filter material beneath a layer of drainage material at the water outlet.

Because water reduces the effective stress and consequently the shear strength of the soil and applies stresses when water is moving through the soil, it has a significant impact on the stability and erosion resistance of both natural and artificial soil structures.

The stability of structures erected on or in soil is therefore improved by draining the water out of the soil structures. The soil must be drained, nevertheless, in a controlled manner. Particles within the soil skeleton or near the surface cannot be eroded by hydraulic gradients and the flow forces that result. This is ensured for natural soils by restricting the hydraulic gradient. Filter zones built into the soil structure are used to control erosion for man-made constructions.

Design of Filters

The following six factors are taken into account when designing filter materials:

(a) Filter ability
(b) Internal stability
(c) Self-healing
(d) Material segregation
(e) Drainage capacity
(f) Material durability

Filter Ability

To design soil filters, the U.S. Army Corps of Engineers (Huang, 2004) set out some criteria. These criteria are based on the particle sizes that, according to the particle size distribution curve, correspond to specific weight percentages of the protected soil and the filter material.

Clogging criterion:
To ensure that the protected soil does not clog the larger particles of the filter, the following criterion must be satisfied by the relative sizes;
D_15,filter/D_85,soil ≤ 5.0

Permeability criterion:
To ensure that water passes through the filter system without building up excess pressure, the following criterion is recommended;
D_15,filter/D_15,soil ≥ 5.0

Additional criterion:
U.S. Army Corps of Engineers also recommends the following additional criterion;
D_50,filter/D_50,soil ≤ 25.0

Based on the above equations, one can design a satisfactory filter system when the particle size distributions of the relevant soil samples are available.

Bertram (1940) proposed the criterion D_15,filter/D_85,soil ≤ 6 for soil filters based on laboratory investigations. This filter criterion was later modified to D_{15coarse-side,filter}/D_{85fine-side,soil} ≤ 4.

A drainage criterion of D_{15fine-side,filter}/D_{85coarse-side,soil} ≥ 4 was added by Terzaghi and Peck (1948)(See figure 3).

Internal Stability

When a filter material is internally stable, it prevents small soil particles from moving due to the forces of water flow. Even for water flow at high (>>1) hydraulic gradients, as happens at a fracture in the sealing zone of an embankment, all soil particles ought to stay in place. For instance, Kenney & Lau (1985) provide a useful definition of internal stability as the capacity of a granular material to avoid the loss of its own microscopic particles as a result of disturbing forces like seepage and vibration.

The filter material’s gradation curve is split into two curves at a chosen grain diameter (dS), gradation curves for the sections finer and coarser than dS, respectively, in this technique, also known as the “retention ratio criteria.” The Terzaghi filter criterion is used to compute the retention ratio (RR) for the two gradation curves: RR = D_15,filter/D_85,soil. This is repeated for various dS values. If all grains meet the criteria RR  ≤  7-8 rounded grains or RR  ≤ 9-10 for angular grains, they are all regarded as stable.

Self Healing

Self-healing means that when there is water flow, cracks that could develop in the filter zone owing to things like differential settlement, etc., close instead of remaining open. Therefore, cohesion cannot exist in the filter material. By limiting the amount of non-plastic (I_P < 5%) fines to under 5%, this is ensured. The sand-castle test (Vaughan & Soares 1982) attests to the filter material’s compliance with the self-healing specifications.

Material Segregation

The filter zone can no longer serve its purpose of preventing fine particles from moving from the core to the filter zone or within the filter zone when the filter material segregates, meaning that the coarser particles separate from the finer particles. This is because the segregated coarse-grained components do not form a filter to the adjacent materials. Therefore, it is necessary to prevent the segregation of filter elements. Most experts concur that a high sand content and a small maximum grain size lessen segregation.

Drainage capacity

The Terzaghi criterion D_15,filter/D_85,Soil ≥ 4 still applies and Sherard recommends D_15,filter ≥ 0.2 mm.

Material Durability

Standard tests like the Los Angeles abrasion test (ASTM C535) or the wet and dry strength variation (typical limit 35%) are frequently used to examine the durability of filter materials. For significant dam structures, mineralogical and chemical analysis of the dam material is suggested This can show whether the substance contains inclusions of (i) swellable clay minerals or (ii) water-soluble minerals, such as gypsum or carbonate rocks.

In addition to dissolving, more recent materials can also re-cement at particle interactions and produce real cohesiveness. Dam filter materials containing carbonate and sulfide should be handled carefully.

Conclusion

The stability and erosion resistance of both natural and artificial soil structures are significantly influenced by water. The stability of the soil structure is increased by draining the water out of it. The soil must be drained, nevertheless, in a controlled manner to prevent erosion. Filter materials positioned inside or on top of the soil structures are used to achieve this.

Filter materials must possess specific properties that are detailed by filter criteria, which has undergone significant progress in recent decades. These filter criteria currently consist of six distinct sections, and each of these criteria has been discussed in this article.